Home /
Expert Answers /
Advanced Math /
let-a-4-2-8-2-7-4-8-4-8-the-eigenvalues-of-the-real-symmetric-matrix-a-are-lambda-1-1-pa432
(Solved): Let A=[[-4,2,8],[2,-7,4],[8,4,8]]. The eigenvalues of the real symmetric matrix A are \lambda _(1)=1 ...
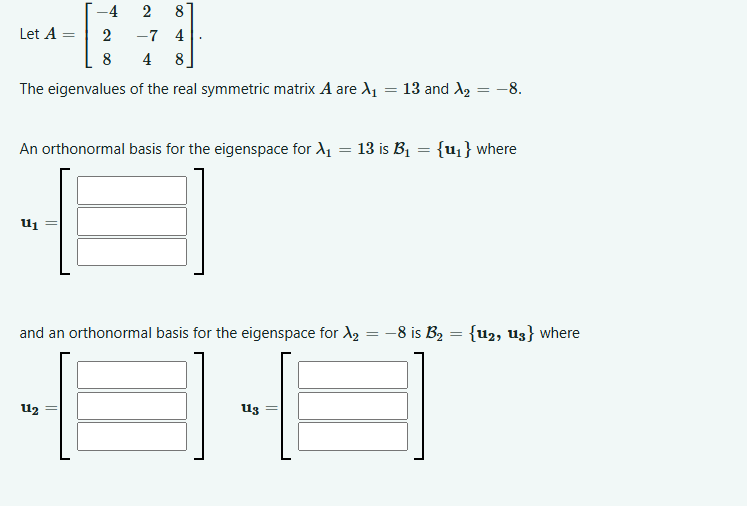
Let A=[[-4,2,8],[2,-7,4],[8,4,8]].
The eigenvalues of the real symmetric matrix A are \lambda _(1)=13 and \lambda _(2)=-8.
An orthonormal basis for the eigenspace for \lambda _(1)=13 is B_(1)={u_(1)} where
u_(1)=[[,|],[,|]]
and an orthonormal basis for the eigenspace for \lambda _(2)=-8 is B_(2)={u_(2),u_(3)} where
u_(2)=[[,|],[,|]],u_(3)=[]