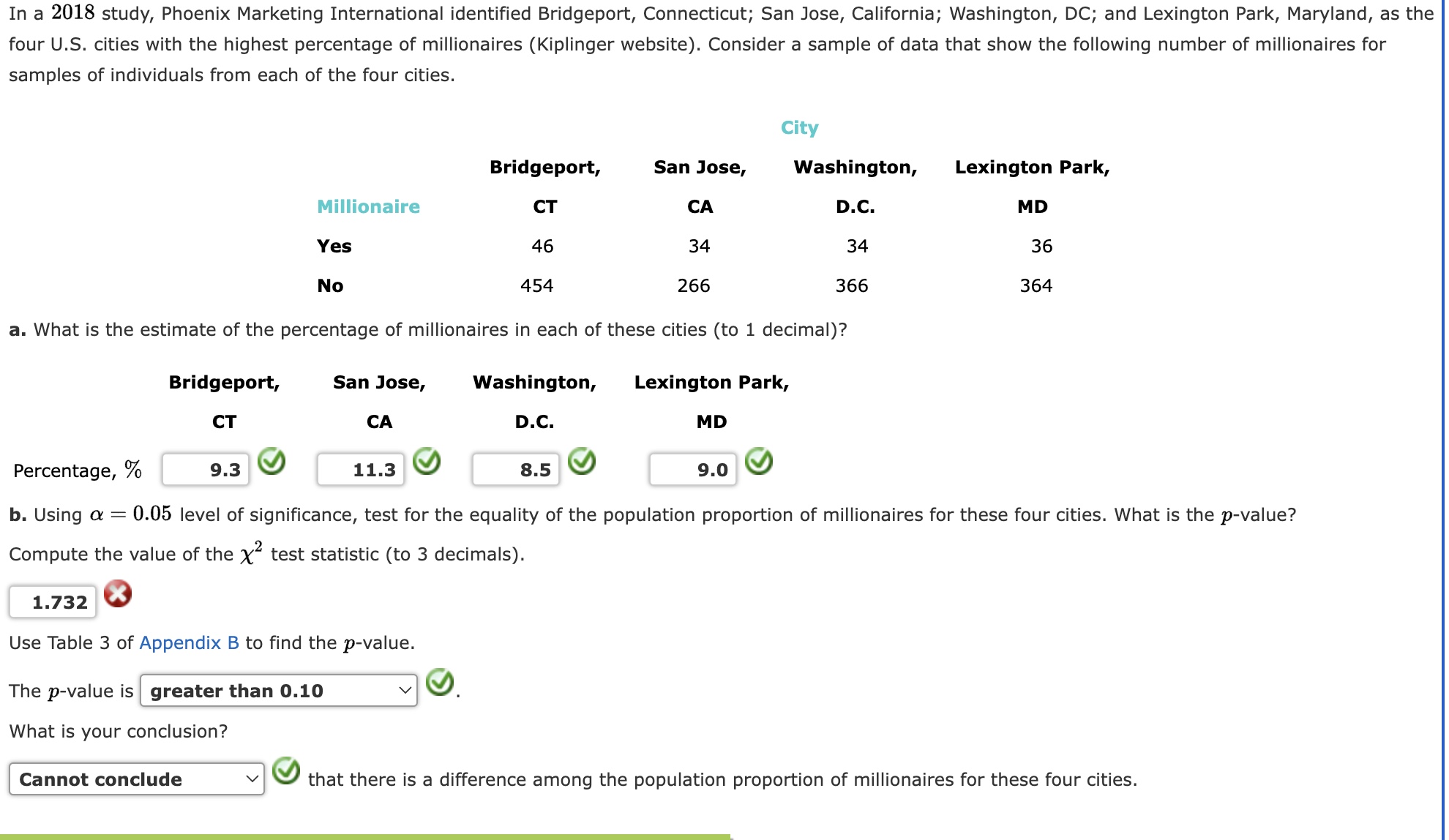
(Solved): In a 2018 study, Phoenix Marketing International identified Bridgeport, Connecticut; San Jose, Calif ...

In a 2018 study, Phoenix Marketing International identified Bridgeport, Connecticut; San Jose, California; Washington, DC; and Lexington Park, Maryland, as the four U.S. cities with the highest percentage of millionaires (Kiplinger website). Consider a sample of data that show the following number of millionaires for samples of individuals from each of the four cities. a. What is the estimate of the percentage of millionaires in each of these cities (to 1 decimal)? Bridgeport, CT San Jose, CA Washington, D.C. Lexington Park, MD Percentage, \% b. Using \( \alpha=0.05 \) level of significance, test for the equality of the population proportion of millionaires for these four cities. What is the \( p \)-value? Compute the value of the \( \chi^{2} \) test statistic (to 3 decimals). Use Table 3 of Appendix B to find the \( p \)-value. The \( p \)-value is What is your conclusion? that there is a difference among the population proportion of millionaires for these four cities.